Embark on an algebraic adventure with Chapter 2 Glencoe Algebra 1, where we delve into the fascinating world of linear equations and inequalities. Prepare to sharpen your problem-solving skills and discover the practical applications of these concepts in our everyday lives.
In this chapter, we will explore the fundamental principles of linear equations, mastering various techniques to solve them. We will also investigate systems of linear equations, uncovering their significance and unraveling their solutions. Additionally, we will delve into the realm of inequalities, understanding their types and honing our skills in solving them.
Chapter Overview
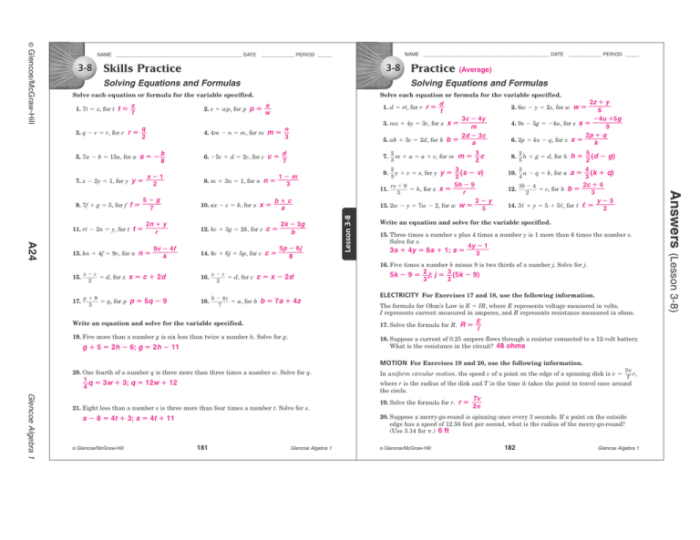
Chapter 2 of Glencoe Algebra 1 introduces students to the fundamental concepts of expressions, equations, and inequalities. These foundational building blocks are crucial for understanding more advanced mathematical concepts in later chapters.
This chapter covers the following key concepts:
- Expressions and their evaluation
- Solving equations using various methods
- Solving inequalities and graphing their solutions
Linear Equations
Linear equations are mathematical equations that represent a straight line when graphed. They are written in the form y = mx + b, where m is the slope of the line and b is the y-intercept.
Linear equations can be solved using a variety of methods, including substitution, elimination, and graphing. In this chapter, we will discuss each of these methods in detail and provide examples of how to solve linear equations.
Solving Linear Equations Using Substitution
Substitution is a method of solving linear equations that involves replacing one variable with an expression that is equivalent to that variable.
For example, to solve the equation 2x + 5 = 11, we can substitute 3 for x:
2(3) + 5 = 11
6 + 5 = 11
11 = 11
Since the equation is true, we know that x = 3 is the solution to the equation.
Systems of Linear Equations
Systems of linear equations are a set of two or more linear equations that are solved simultaneously. They are used to model real-world situations involving multiple variables and relationships.
Solving systems of linear equations is essential in various fields, including science, engineering, economics, and daily life. They help us find the values of unknown variables that satisfy all the equations in the system.
Methods for Solving Systems
There are several methods for solving systems of linear equations, each with its advantages and disadvantages.
- Graphing:This method involves plotting the graphs of each equation and finding the point(s) of intersection. However, it can be challenging to find precise solutions, especially for complex systems.
- Substitution:This method involves solving one equation for a variable and substituting its expression into the other equations. It is straightforward but can lead to complex algebraic manipulations.
- Elimination:This method involves adding or subtracting the equations to eliminate one variable and solve for the other. It is generally more efficient than substitution for systems with two or three variables.
Sample Systems
Let’s consider the following system of equations:
x + 3y = 12
x
y = 1
Using the elimination method, we can add the two equations to eliminate y:
(2x + 3y) + (x
- y) = 12 + 1
- x + 2y = 13
Now, we can solve for x:
- x = 13
- 2y
x = (13
2y) / 3
Substituting this expression for xinto the second equation, we can solve for y:
((13
- 2y) / 3)
- y = 1
y = 2
Finally, we can substitute the value of yback into the expression for xto get:
x = (13
2(2)) / 3
x = 3
Therefore, the solution to the system is x= 3 and y= 2.
Inequalities
Inequalities are mathematical statements that compare two expressions. They are used to represent relationships between numbers that are not equal.
Inequalities are represented on a number line using symbols such as <, >, ≤, and ≥. These symbols indicate the direction of the inequality.
Chapter 2 of Glencoe Algebra 1 introduces us to the concept of solving equations. To get a better grasp on this, you might want to take a look at the repaso de a estudiar quiz . This quiz will test your understanding of the concepts covered in Chapter 2 and help you identify areas where you need more practice.
So, once you’ve familiarized yourself with the chapter, be sure to give the quiz a try to reinforce your learning.
Types of Inequalities
There are different types of inequalities, including:
- Linear inequalities: These inequalities involve linear expressions. For example, 2x + 1 > 5.
- Absolute value inequalities: These inequalities involve absolute value expressions. For example, |x – 3|< 2.
- Compound inequalities: These inequalities involve two or more inequalities connected by the words “and” or “or”. For example, x > 2 and x< 5.
Solving Inequalities, Chapter 2 glencoe algebra 1
To solve inequalities, we use the same operations that we use to solve equations. However, we need to be careful when multiplying or dividing by negative numbers.
When we multiply or divide both sides of an inequality by a negative number, we need to reverse the direction of the inequality.
Applications of Linear Equations and Inequalities: Chapter 2 Glencoe Algebra 1
Linear equations and inequalities are powerful tools that can be used to solve a wide variety of real-world problems. In this section, we will explore some of the most common applications of these concepts.
One of the most common applications of linear equations is to model relationships between two variables. For example, the equation y= 2 x+ 1 models the relationship between the input variable xand the output variable y. This equation tells us that for every increase of 1 in the value of x, the value of ywill increase by 2.
Linear equations can also be used to solve problems involving rates and proportions. For example, if a car is traveling at a speed of 60 miles per hour, then the distance traveled by the car in thours is given by the equation d= 60 t.
Inequalities are also useful for modeling real-world problems. For example, the inequality x> 5 models the set of all numbers that are greater than 5. This inequality can be used to solve problems involving constraints or limits.
Word Problems
Here are some examples of word problems that can be solved using linear equations and inequalities:
- A farmer has 100 feet of fencing to enclose a rectangular plot of land. What is the maximum area that the farmer can enclose?
- A store is selling apples for $0.50 each and oranges for $0.75 each. How many of each type of fruit must the store sell to make a total of $100?
- A company is manufacturing two types of products, A and B. Product A requires 2 hours of labor to produce, and product B requires 3 hours of labor to produce. The company has a total of 100 hours of labor available per day.
How many units of each product can the company produce each day?
These are just a few examples of the many ways that linear equations and inequalities can be used to solve real-world problems. By understanding these concepts, you will be able to apply them to a wide variety of situations.
FAQ Explained
What is a linear equation?
A linear equation is an equation of the form Ax + B = C, where A, B, and C are constants and x is the variable.
How do you solve a system of linear equations?
There are several methods to solve systems of linear equations, including graphing, substitution, and elimination.
What is the difference between an equation and an inequality?
An equation is a statement that two expressions are equal, while an inequality is a statement that two expressions are not equal.